©Copyright 2018 GEOSCIENCE RESEARCH INSTITUTE
11060 Campus Street • Loma Linda, California 92350 • 909-558-4548

CARBON-14 CONTENT OF FOSSIL CARBON
Paul A. Giem
Loma Linda, California
WHAT THIS ARTICLE IS ABOUT
This article reviews the theoretical basis for expecting the presence of carbon-14 in Pliocene to Cambrian carbon from certain creationist viewpoints, and for expecting its absence from a viewpoint proposing a long age of life on Earth. The relevant experiments are discussed. Several conclusions emerge: 1) There is measurable carbon-14 in material that should be "dead" according to standard evolutionary theory; 2) machine error can be eliminated as an explanation for this carbon-14 on experimental grounds; 3) nuclear synthesis of this carbon-14 in situ can be eliminated on theoretical grounds; 4) contamination of fossil material in situ is unlikely but theoretically possible, and is a testable hypothesis; 5) contamination during sample preparation is a significant problem but theoretically soluble; 6) residual activity is most likely indicated by the present data, and if correct, would eliminate an age greater than approximately 100,000 years for life on Earth; and 7) additional experimental evidence cannot eliminate either a short or a long age of life on Earth, but can provide evidence tending to discriminate between the two.
CLASSIFICATION OF MAJOR THEORIES OF EARTH HISTORY
This paper deals with the presence of carbon-14 in fossil material and its implications for theories of the age of life on Earth. [1] For our purposes these theories can be divided into roughly three categories:
-
- Theories which assume that life has been on Earth for 1-4 billion years include mechanistic evolution, theistic evolution, multiple creations/progressive creation, and ruin-and-restoration theories.
- Theories which assume that life has been on Earth for less than 100,000 years and that radiometric decay constants have remained constant during that time include various forms of special creationism. These include those placing the Flood at the time indicated by the Masoretic text of Genesis 11 (4,300-4,500 years ago), those dating the Flood by the Septuagint (5,500 years ago), and those placing the Flood at a somewhat more remote time (usually about 10,000-20,000 years ago).
- Theories assuming that life has been on Earth for less than 100,000 years and that radiometric decay constants have not remained constant during that time include various forms of special creationism which may be quite similar to those mentioned in the second category, except for their view regarding decay constants. [2]
The predictions of the third category of theories regarding carbon-14 in fossil carbon (carbon from such sources as coal, oil, natural gas, wood, or bone) usually match those of the first category, although they are not logically required to do so. In fact, unless there are some constraints on how much radiometric constants may vary, the third category of theories cannot make any predictions whatever. In this paper we are concerned with theoretical predictions and their match with experimental evidence. Since the third category has difficulty making any predictions regarding carbon-14 in fossil carbon, it will be ignored here, not because we know it to be wrong, but because it is untestable.
LONG-AGE THEORIES PREDICT NO CARBON-14 IN GEOLOGICALLY OLD SAMPLES
In the first category — long-age theories —, some rather definite predictions can be made about samples that are assigned an age greater than 100,000 years. No one assumes that the concentration of carbon-14 in ordinary carbon ( 14C/C ratio) in the biosphere has ever been more than 10× the present 14C/C ratio. One can accordingly establish a reasonable upper limit of 0.0056 percent modern carbon (pmc) for the 14C/C ratio in a 100,000-year-old specimen. Every 57,100 years the 14C/C ratio decreases by a factor of 1,000. A 200,000-year-old specimen should have a present 14C/C ratio of 0.000 000 031 pmc or less. By the time we get back to 300,000 years, a sample should have less than one atom of carbon-14 in a gram of carbon as residual activity. [3] This means that one million-year-old samples, or 350 million-year-old samples, should have no residual radiocarbon.
Explanations of measured radiocarbon in an old sample that are consistent with long-age theories might include carbon-14 created there by nuclear synthesis, carbon-14 from elsewhere contaminating the sample (either in the ground or during sample preparation), or machine error (the measuring device indicating the presence of carbon-14 in the sample when in fact there is none). These possible sources of error will be discussed below.
MOST SHORT-AGE CONSTANT-DECAY MODELS PREDICT A SMALL AMOUNT OF CARBON-14 (0.6 TO 0.005 PMC) IN GEOLOGICALLY OLD SAMPLES
The predictions of the second category of theories, which we shall call short-age constant-decay theories, are not as clear-cut. There is general agreement among short-age theories that the Paleozoic and Mesozoic sediments were deposited by the Flood, and are thus contemporaneous. Some would have sediments up to the Pliocene also deposited by the Flood, while others would have the Pliocene and possibly other Cenozoic sediments be immediately post-Flood. The date of the Flood would vary from theory to theory, although usually by less than 20,000 years). In addition, there are questions about how much carbon-14 was in the earth at Creation, how much carbon-14 was being formed per year before the Flood, and how much ordinary carbon was in the biosphere at the time the Flood started.
With the simplest case, we will assume that Earth was created in equilibrium with respect to carbon-14, and that the cosmic ray flux, Earth's magnetic field, and distribution of nitrogen in the atmosphere before the Flood were all essentially the same as today. Then we can assume that the amount of carbon-14 in the biosphere was the same as it is today. A short-age Flood model requires that this carbon-14 would have been diluted in a pool of ordinary carbon (carbon-12 and carbon-13) vastly greater than that of today. How much greater that pool was would be based on the amount of existing fossil carbon. Certainly the carbon from all the coal in Flood strata, probably all the oil and possibly the natural gas, [4] and an unknown percentage of all the limestone would have been in the biosphere. Fossil shells should have been in the biosphere, but there could have been a reservoir effect so that they would have less carbon-14 than expected. Amorphous or crystalline calcium carbonate may or may not have been in equilibrium with the biosphere. Therefore, to find the pre-Flood pool of ordinary carbon, one would need to add all the coal, oil, and possibly natural gas reserves, and some percentage of the world deposits of limestone. The 14C/C ratio expected before the Flood would then be the present one divided by the ratio of fossil carbon to carbon in the biosphere.
The best estimates I have seen for carbon in various reserves were collected by Brown (1979). More recent estimates (for example, Scharpenseel and Becker-Heidmann 1992) agree within a factor of 2. Brown's estimate for carbon in the modern biosphere was 3.9×10 13 metric tons, for fossil organic carbon 6.8×10 15 metric tons, and for sedimentary carbonate 1.3×1016 metric tons. Accordingly, the pre-Flood reservoir of ordinary carbon would have been some 180-510× as much as at present. This estimate could easily be in error by a factor of 2 or so in either direction. This would affect the denominator of the 14C/C ratio and thus decrease this ratio in the pre-Flood era by some 200-500× and possibly up to 1000× compared to the modern era. Based on these considerations, my best estimate would be about 200×, but 100-400× seems reasonable.
The numerator of the 14C/C ratio could also have been different before the Flood. Some of the factors that could reasonably affect the numerator are the cosmic ray flux and the amount of carbon-14 existing at the time of creation. If one assumes that at creation there was no carbon-14 in the biosphere, and that the Flood was 1656 years after creation (the shortest reasonable time), then at the time of the Flood carbon-14 would have built up in the biosphere to 18% of its equilibrium value, based on a constant production rate for carbon-14. In addition, if the magnetic field was at maximum reasonable strength, the production of carbon-14 would have been reduced by approximately 75% (Brown 1979). A stronger magnetic field would seem to be a very reasonable assumption. Finally, a vapor canopy might have reduced the production of carbon-14 by an unknown amount, although a physically stable vapor canopy would probably have had a minor effect that may be ignored for our purposes.
It is not unreasonable to postulate a very low, non-equilibrium total amount of carbon-14 in the original atmosphere. This is not likely to be explained by the theory that there was no carbon-14 immediately after creation because creation was perfect. That theory would imply that there were no other radioactive isotopes immediately after creation. Other radioactive isotopes such as potassium-40 are in the biosphere now, and they were probably in the biosphere at the time of the Flood, and also at creation. However, if one assumes that Earth's matter existed before creation, there is the possibility that the primeval atmosphere contained primarily water vapor and was devoid of nitrogen, in which case the production of carbon-14 from cosmic rays would be markedly reduced. So a minimal concentration of carbon-14 at creation week cannot be ruled out.
Finally, time since the Flood must be factored into any model for carbon-14 dating. If one follows a Masoretic chronology, there would be a reduction in the carbon-14 in pre-Flood samples of 41-42% due to the time since the Flood. For a Septuagint chronology, the reduction would be about 50%, and for gap theories the reduction would be substantially larger. Putting the Flood at the date proposed by Aardsma (1991) — 12,000 B.C. — would reduce the amount of carbon-14 by 82%. Putting it at 57,100 years ago would reduce it by 99.9%. At this point I will ignore theories that place the Flood more than about 20,000 years ago (and would reduce the concentration of carbon-14 by >91%), although it must be acknowledged that these theories cannot be logically excluded from consideration.
The various factors that would reduce the pre-Flood 14C/C ratio in the biosphere are not entirely independent of each other. One can propose a Flood at 20,000 years ago, but if one expands the post-Flood chronology, then it seems illogical to assume a short chronology for the time between creation and the Flood. Increasing the time between creation and the Flood provides more time for carbon-14 to equilibrate and lessens the apparent aging effect of starting with little or no carbon-14 at creation. Thus it is not accurate to take all the reduction factors and simply multiply them together. The same is true, although to a lesser degree, for models based on the Septuagint. Finally, if one assumes that some of the carbon in the Phanerozoic fossil record came from comets or meteorites, the reduction in the pre-Flood 14C/C ratio caused by a larger pre-Flood biomass must be decreased by the proportion of "fossil" carbon that did not come from the earth.
We will now make some estimates using the above assumptions. For example, suppose we start with a Masoretic chronology, a stronger pre-Flood magnetic field, and a negligible amount of carbon-14 at Creation. Then a reasonable first approximation for the expected measured 14C/C ratio of fossils buried in the Flood is the reduction due to biomass, multiplied by the reduction due to the magnetic field, multiplied by the reduction due to non-equilibrium conditions at the beginning, multiplied by the reduction due to the passage of time since the Flood. My best a priori estimate of the numbers would be 1/200 × 1/4 × 1/5 × 60%, or 1/6,667, which would correspond to 0.015 pmc measured. It could be as low as 1/4 of that if our estimates of fossil carbon are low and the carbon in limestone was in equilibrium with the biosphere, [5] although experimental evidence (see below) indicates that dolomite was not in equilibrium with the biosphere and suggests that most of the limestone was also not in equilibrium with the biosphere. A more likely lower limit would be 1/13,333, or 0.0075 pmc. A reasonable upper limit would be 1/100 (low estimate of reduction due to biomass) × 60%, or 0.6 pmc. Much of the spread in the upper limit is due to differences in assumptions regarding the pre-Flood magnetic field, assumptions that are presently untestable. A Septuagint chronology would predict roughly the same numbers. The decrease due to a longer time since the Flood would be almost exactly offset by an increase due to more time between creation and the Flood. The only change would be that the upper limit would drop to 0.5 pmc.
Ancient flood models probably should not use factors for non-equilibrium conditions before the Flood. Therefore the model proposed by Aardsma (1991) should predict a most likely concentration of 1/200 × 1/4 × 18%, or 0.0225, with a lower estimate of 0.01125 (or 0.01) pmc and an upper estimate of 0.18 pmc. At 21,000 years the estimate should be roughly 0.01 pmc with a lower limit of 0.005 pmc and an upper limit of 0.08 pmc. Calculations could be made for other models, but these calculations give one a feel for the predictions that can be expected from various models. It is of interest that there is so little variation in the predictions for the lower limit for present-day measurements of pre-Flood fossil carbon among the major models.
CARBON-14 IS FOUND CONSISTENTLY IN GEOLOGICALLY OLD SAMPLES
When carbon-14 dating was first developed, the level of carbon-14 was measured by counting the decay of carbon-14 atoms in a given sample (decay counting). This was associated with a high background count, which, under most circumstances, swamped the low levels of carbon-14 expected by short-age constant-decay theories noted above. It also necessitated having a control counter, which would be filled with supposedly "dead" carbon. Any small residual amount of carbon-14 in the "dead" carbon would not be detected, because the method guarantees that any residual would be subtracted out. A possible exception would be if one used truly non-fossil carbon which was known to contain no residual carbon-14 for the comparison. As far as I know, such an experiment has never been reported, and it is difficult to imagine it being done by someone who did not consider short-age constant-decay theories a live option.
A method called isotope enrichment might be able to find carbon-14 in fossil material even given the above difficulties. This method involved concentrating the carbon-13 and carbon-14 in a specimen by fractional distillation of carbon monoxide. The fraction of carbon-14 in a specimen is increased, making it measurable using standard decay-counting techniques. This method could theoretically detect carbon-14 in geologically old specimens, since, for example, carbon dioxide from anthracite coal can be compared with enriched carbon dioxide from anthracite coal.
The experiment in question was done at least three times (Grootes et al., 1975). The first time, the results on anthracite were 0.023±0.011 pmc. Grootes et al. believed that there was contamination in the system. They made some changes in the process and repeated the experiment two more times, getting 0.0072±0.0096 pmc, and 0.0062±0.0038 pmc. If the last two results are combined statistically, they give 0.0064±0.0035 pmc, which is not statistically different from zero. This particular method has fallen out of favor. The reason for this is not documented in the literature, but probably was due at least in part to the fact that it involved a difficult, time-consuming fractional distillation followed by a time-consuming process of counting decays.
In the late 1970s a new method of measuring carbon-14, called AMS, or Accelerator Mass Spectrometry dating, was developed. This involved directly counting the carbon-14 atoms, using a tandem accelerator. Since the atoms are first negatively charged, most of the interference from nitrogen-14, which is much more common than carbon-14 but does not easily take a negative charge, is eliminated. In addition, each atom is accelerated by a very high voltage, and several tests can be done to make sure that we are in fact measuring carbon-14 instead of some interfering isobar, or cosmic rays. Theoretically, the machine should have zero machine background, which makes it ideal for attempting to detect carbon-14 in geologically old specimens. [6]
If one defines machine background as carbon-14 equivalent counts without a sample in place, the predictions of zero background turn out to be largely correct. Schmidt et al. (1987) were able to run their machine with an empty aluminum target holder without finding any atoms of carbon-14 in a 30-minute run, which would be equivalent to >90,000 radiocarbon years (<0.0014 pmc) if they had had a standard current of ordinary carbon. Van der Plicht et al. (1995) found an equivalent age of >100,000 radiocarbon years, and Kirner et al. (1995) obtained an equivalent age of >104,000 years.
Careful experimental technique is necessary. Some experiments did show small amounts of carbon-14 in the machine blanks. Donahue et al. (1984) found carbon-14 atoms equivalent to 0.08 pmc with an empty target holder. Kitagawa et al. (1993) obtained 0.03 pmc. Beukins et al. (1992) did better (0.015±0.007 pmc). Apparently with more careful technique one can reduce the machine background to negligible levels, as noted in the preceding paragraph.
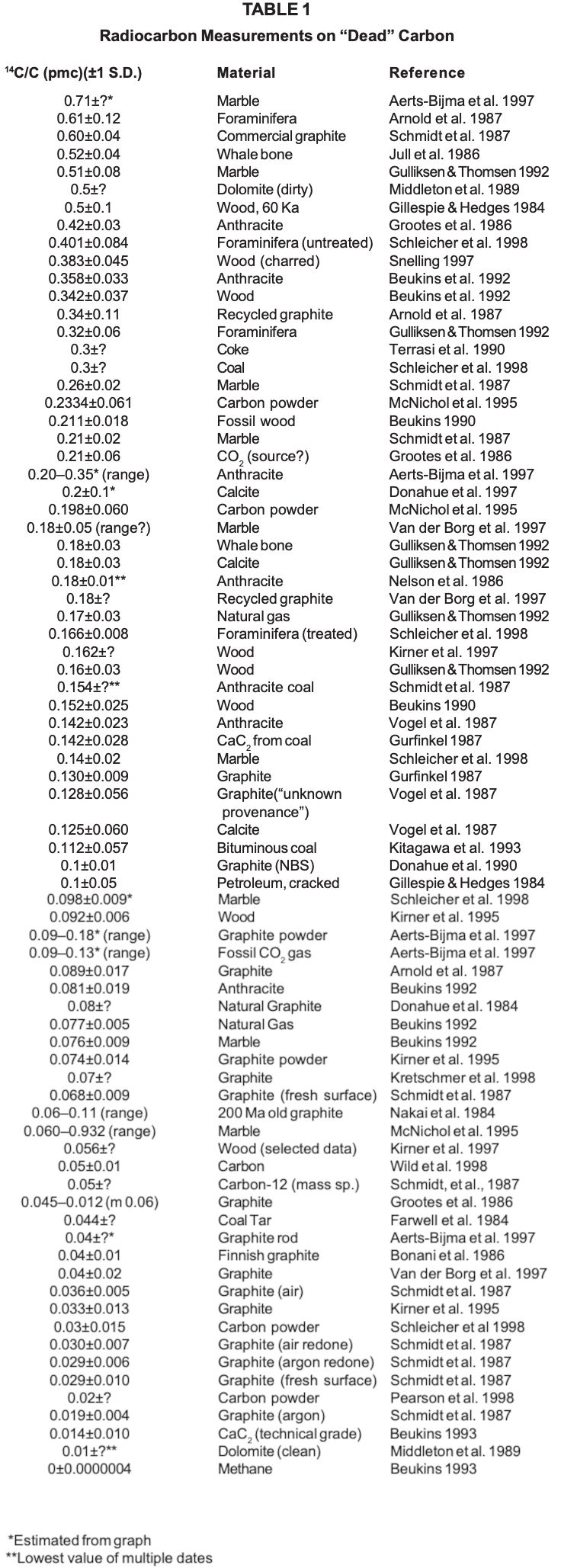
However, as one can see from Table 1 (p 14-15), carbon samples have not matched the best results from machine blanks. There is some residual carbon-14 in even the most carefully prepared samples, so that the article by Schmidt et al. was entitled "Early expectations of AMS: greater ages and tiny fractions. One failure? — one success." As can be seen from Table 1, further experiments have continued to find carbon-14 in supposedly "dead" carbon, raising the question as to how to explain this carbon-14.
Short-age constant-decay theories predict that fossil carbon should contain a small amount of carbon-14, and one explanation of the above data is that one of these short-age theories is correct. But there are other possible explanations. The obvious ones are machine background which only happens when carbon-12 and/or carbon-13 are in the machine, contamination of the source deposits in the ground ( in situ), contamination of the samples with modern carbon during sample processing, or the creation of carbon-14 in situ by nuclear reactions.
MACHINE BACKGROUND IS NOT AN ADEQUATE EXPLANATION
The hypothesis that machine background can account for this carbon-14 has been universally rejected by researchers in the field, and for good reason. Any atom which is counted as carbon-14 must pass at least 3, and sometimes 4 tests. First, it must pass through the accelerator. Remember that there is no difference between these experiments and those that have an empty sample holder, except the sample. So any difference between the experiments must be explained by something which goes through the accelerator. But after the accelerator there is a magnet which separates the beam by its charge-to-momentum ratio. So any ion which strikes the detector in the first place must have the charge-to-momentum ratio of carbon-14. Second, the amount of energy lost creating ions in a defined thickness of semiconductor material is measured, and only one narrow range of values is consistent with carbon-14 that has traveled through the accelerator. Third, the total energy, measured by ions created in a thickness of semiconductor thick enough to stop the carbon-14, is measured. This again results in a narrow range of acceptable values consistent with carbon-14.
These three tests are enough by themselves to uniquely identify carbon-14 and to distinguish it from nitrogen-14, carbon-13 with hydrogen, carbon-12 with two hydrogens or with deuterium, two lithium-7 atoms, other molecular species, or cosmic rays. However, in some experiments, most notably Bonani et al. (1986), the time of flight of each particle between the ion stripper in the middle of the tandem accelerator and the detector was also measured, and it was also consistent with carbon-14 and not with other molecular species. Thus one can be quite sure that the atoms that are being detected are indeed carbon-14. [7]
NUCLEAR SYNTHESIS OF CARBON-14 IN SITU IS NOT AN ADEQUATE EXPLANATION
The next explanation that might be made is that these carbon-14 atoms are created by nuclear reactions while the sample is in the ground. This is highly improbable. Zito et al. (1980) calculated that groundwater in granite could possibly have carbon with a carbon-14 concentration of 0.00266 pmc. Florkowski et al. (1988) corroborated their calculations. If one reworks the calculations using oil, one comes up with 2.7×10 -8 pmc (Giem 1997a, p 186-187). This is well below the range capable of explaining the above experiments.
One can hypothesize that neutrons were once much more plentiful than they are now, and that is why there is so much carbon-14 in our experimental samples. But the number of neutrons required must be over a million times more than those found today, for at least 6,000 years; and every 5,730 years that we put the neutron shower back doubles the number of neutrons required. Every time we halve the duration of the neutron shower we roughly double its required intensity. Eventually the problem becomes insurmountable. In addition, since nitrogen-14 captures neutrons 110,000 times more easily than does carbon-13, a sample with 0.000 0091% nitrogen should have twice the carbon-14 content of a sample without any nitrogen. If neutron capture is a significant source of carbon-14 in a given sample, radiocarbon dates should vary wildly with the nitrogen content of the sample. I know of no such data. Perhaps this effect should be looked for by anyone seriously proposing that significant quantities of carbon-14 were produced by nuclear synthesis in situ.
CONTAMINATION IN SITU EXPLAINS SOME, BUT PROBABLY NOT ALL, THE RESULTS
Contamination in situ is sometimes used to explain the persistent residual carbon-14 found in these experiments. Some experiments virtually demand this explanation as at least a contribution to the results obtained. For example, Schleicher et al. (1998) note that relatively untreated foraminifera gave 0.401±0.084 pmc, whereas foraminifera treated with various methods for removal of contamination gave a smaller 14C/C ratio, reaching 0.166±0.008 pmc when using a purification procedure including 30% H 2O2 and 15 min of ultrasonic treatment, and attachment to the carbonate system wet. This is highly unlikely (p<0.001) to be due to chance. However, it appears that the best data on fossil carbon with a published standard deviation, other than Beukins (1992), Kirner (1995), and Beukins (1993), all cluster at about 0.15 pmc and are not statistically different from one another (see Figure 1). It is difficult to imagine a natural process contaminating wood, whalebone, petroleum and coal, all to roughly the same extent. It is especially difficult to imagine all parts of a coal seam being contaminated equally.
However, contamination in situ is a more likely consideration than is nuclear synthesis in situ or machine error. One could evaluate the contamination hypothesis by carefully testing different samples, such as coal from different parts of a seam, and from different depths, coke, coal tar, petroleum, and wood. If all sources come out with similar amounts of carbon-14, we can be reasonably sure that contamination in situ is not a good explanation for the observed amount of carbon-14. The available data suggest this to be the case, but do not quite prove it. On the other hand, if we are consistently able to get significantly lower 14C/C ratios with some samples of pre-Pleistocene fossil carbon than with others, this suggests that the differences between the 14C/C ratios in the various samples may reasonably be explained by contamination in situ .
CONTAMINATION DURING SAMPLE PROCESSING EXPLAINS SOME, BUT PROBABLY NOT ALL, THE RESULTS
Contamination during sample processing is the most frequent explanation of carbon-14 in samples expected to be "dead" by long-age theories. There is good evidence that contamination during sample processing often occurs, and that some of the carbon-14 found in these samples may be accounted for on this basis. For example, Middleton et al. (1989) measured one dolomite sample that had 0.01 pmc when handled with extreme care, and 0.5 pmc when handled with less care. Van der Borg et al. (1997) noted graphite to have 0.04±0.02 pmc when measured without reprocessing, and 0.18 pmc when tested after recycling. Arnold et al. (1987) reported a graphite having 0.089±0.017 pmc without recycling, and 0.34±0.11 pmc after recycling (statistically significant at p<0.025). Schmidt et al. (1987) analyzed several samples of graphite that varied in 14C/C ratio depending on the care used in preparation. Perhaps most impressively, Schmidt et al. noted a finite "age" (0.05 pmc) for carbon-12 obtained from a Faraday cup in their AMS machine, that was functioning as a mass spectrometer to separate carbon-12 from carbon-14. Contamination during sample processing cannot be ignored. Therefore, results for coal higher than about 1 pmc, which seem to me to be likely to be due to contamination, have not been reported in this paper.
However, contamination is not necessarily inevitable. Some parts of the process do not have to add contamination when done carefully. Beukins (1992) reported anthracite (0.081±0.019), natural gas (0.077±0.005), and marble (0.076±0.009) samples that had essentially the same 14C/C ratio. Since each of these materials is processed differently, these results show that all steps in sample preparation except the reduction step can be done in such a way as to avoid contamination.
It is possible that the iron sometimes used to reduce carbon dioxide to carbon is a source of contamination. In one experiment (Brown et al. 1983), this iron contained carbon with a 1.5 pmc 14C/C ratio.
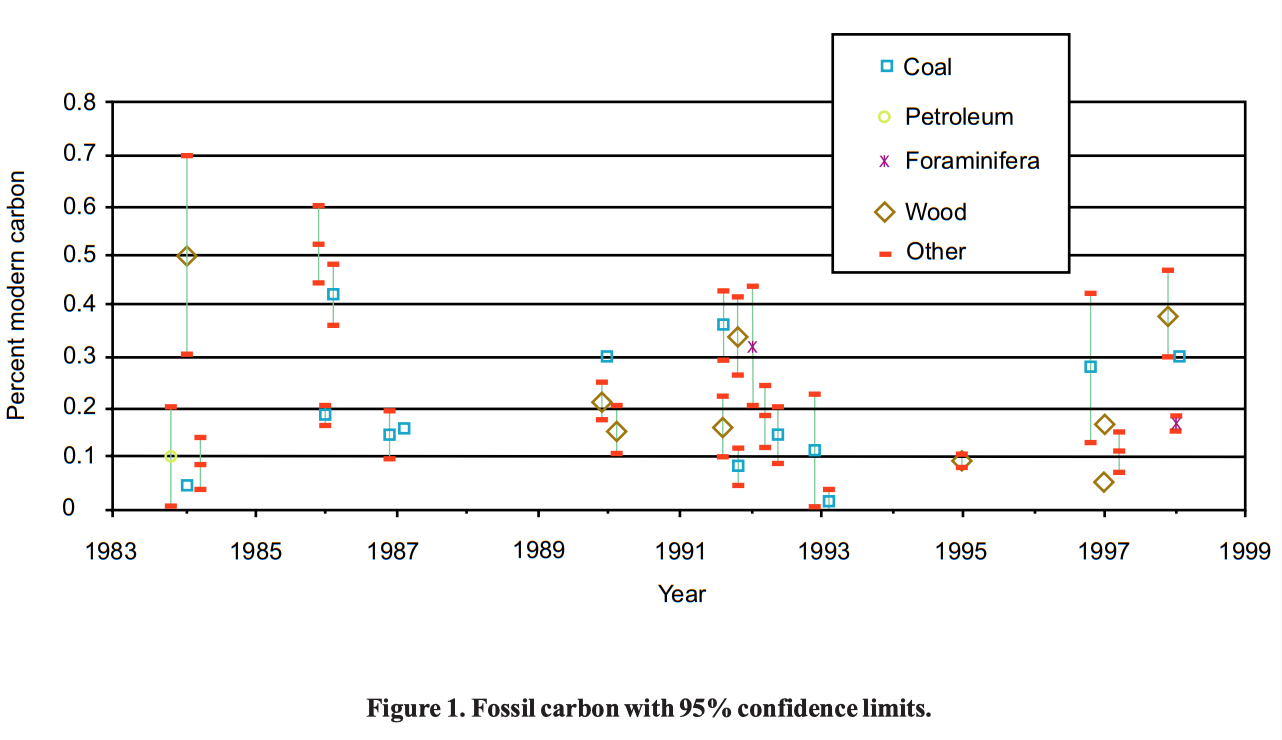
One of the consistent findings in these experiments is that graphite dates older (i.e., has a lower 14C/C ratio) than fossil carbon. Marble and calcite give intermediate results, at least in the best experiments. This is true not only for the overall list but also for several experiments where graphite was directly compared with fossil carbon from various sources (e.g., Schmidt et al. 1987, Aerts-Bijma et al. 1997, Grootes et al. 1986, Vogel et al. 1987). Interestingly, the lowest 14C/C ratio is for dolomite. It has been suggested that the form of the sample influences the amount of contamination. In general, the samples that have to be manipulated the most, and specifically those that require reduction from carbon dioxide to carbon, tend to have higher 14C/C ratios. The dolomite noted above may not be an exception, as it was measured directly as carbon dioxide without being reduced (one of the few experiments to try this technique).
However, it should be noted that some of the graphite samples, and perhaps most of them, come from Finland, where there is Precambrian graphite. In one such case the graphite is specified to be from the bedrock of Finland (Bonani et al. 1986). An equally good hypothesis for the difference between 14C/C ratios in graphite and coal is that Precambrian graphite was not in equilibrium with the pre-Flood biosphere and should have lower residual carbon-14 levels than fossil carbon from the Flood.
RESIDUAL ACTIVITY IS THE MOST LIKELY EXPLANATION FOR CARBON-14 IN PRE-PLEISTOCENE MATERIAL
To summarize, there are two competing theories for the higher 14C/C ratio in fossil material compared to graphite. The first is that there is contamination from the reduction step. The second is that there is more residual carbon-14 in fossil material than there is in graphite. Differences between these theories are testable.
One way to test these competing explanations is to oxidize graphite and run it through the same reduction step as the other materials. This has been done (Van der Borg et al. 1997), and the results were 0.18±0.04 pmc. This result clearly indicates contamination during the reduction process.
Another way to test these explanations would be to use a method that does not require reducing the carbon dioxide from fossil carbon, but instead measures it directly. Measuring the carbon-14 in carbon dioxide directly has been done by Middleton et al. (1989), but I have not found any reports of experiments that compared carbon dioxide from fossil carbon with carbon dioxide from Precambrian or other non-fossil carbon.
Another way to test these explanations is to prepare graphite directly from fossil carbon without first turning the fossil carbon into carbon dioxide. This has been done in at least five experiments. Terrasi et al. (1990) measured coke directly and obtained 0.3 pmc. Beukins et al. (1992) used calcium carbide presumably produced by heating calcium oxide with coal, made acetylene, and cracked it directly to carbon. They obtained a 14C/C ratio of 0.142±0.028 pmc. Gillespie and Hedges (1984) cracked petroleum directly. They obtained a 14C/C ratio of 0.1±0.05. And Farwell et al. (1984) cracked coal tar directly, and obtained a 14C/C ratio of 0.44. These results are compatible with the lowest data from oxidized fossil carbon, and higher than those consistently found in graphite (0.03 pmc). Finally, there are the data of Beukins (1993), which will be discussed below.
Perhaps the best way to test these explanations is to compare Phanerozoic graphite with Precambrian graphite. I am not sure that the graphite samples with the lowest 14C/C ratios are Precambrian, but the assumption is not an unreasonable one (Giem 1997a, p 184). There is one reported experiment that gave a Phanerozoic date for the graphite used (Nakai et al. 1984). Their results ranged from 0.06 to 0.11 pmc, compatible with the results for fossil carbon noted above, and higher than those consistently found in graphite which could be Precambrian. These data argue for residual carbon in fossil carbon (and against contamination during the reduction step).
However, none of the experiments mentioned above were done to test the differences between the various theories. Perhaps the most interesting experiment was reported by Kirner et al. (1997). Part of the background is as follows: R. E. Taylor was aware that short-age constant-decay theories predicted that there should be >0.005 pmc in fossil carbon (Giem 1997a, p 180-187). Taylor believed that he should be able to obtain 14C/C ratios lower than those commonly published, and that could possibly match or even surpass those obtained from graphite. The results his group obtained include several measurements with an average of 0.162 pmc. The lowest value they obtained was 0.056±0.004 pmc. [8] Their conclusions were that the data were best explained as the sum of a constant amount of contamination by modern carbon regardless of sample size, plus a constant proportion of carbon-14 equivalent to 0.12±0.02 pmc. The constant proportion of carbon-14 "could arise if our wood blank was not truly 14C dead either due to a finite age or the result of the presence of residual contamination not removed by chemical treatment." These data argue for the theory of residual carbon-14 in fossil carbon.
One explanation of the data was that it was due to contamination that was gradually getting less with time, as laboratory techniques improved. However, from the graph in Figure 2, it is difficult to detect a trend with time. [9] The lowest values for graphite, and the lowest values for coal, are not the most recent determinations.
Two additional results deserve special attention. Beukins (1993) reports that technical grade calcium carbide, when hydrolyzed to acetylene and cracked, produced graphite with a 14C/C ratio of 0.014±0.010 pmc. This is in contrast to his previously reported 0.142±0.028 pmc (Beukins 1990, also reported in Gurfinkel 1987). Commercial calcium carbide is usually prepared from coal and calcium oxide. If one assumes this is the case here, the 0.014 pmc value reported is possibly the lowest 14C/C ratio for fossil carbon in the literature.
There is also a report of carbon monoxide prepared from methane purified from natural gas (again in Beukins 1993), which was isotopically enriched. The enrichment process apparently was done several times, and the most enriched fraction, in which carbon-14 was theoretically enriched 20,000-fold, had essentially the same 14C/C ratio (uncorrected for enrichment) as the unenriched fraction. Taken at face value, these results suggest that the natural gas had a 14C/C ratio of 0.000 000 0± (4×10 -7), and that either this particular natural gas was not fossil material (see Planetary Sciences Unit 1982), or that short-age constant-decay models are incorrect (as noted above, most reasonable short-age constant-decay models require a 14C/C ratio above 0.005 pmc).
According to one explanation of the data discussed above, all the carbon-14 found in material classified as pre-Pleistocene represents contamination. In that case one has to explain why careful researchers are commonly unable to obtain carbon with less contamination from modern carbon than 1 part in 1,000. One would be encouraged by the data of Beukins (1993), but would have to put it into perspective, especially considering the data of Kirner et al. (1997). Further experiments which might bolster this hypothesis include repeating the experiments of Beukins, using coal from deep underground with minimal opportunity for contamination, and trying isotope enrichment experiments, which may be easier to keep clean.
A second explanation of the data is that material classified as pre-Pleistocene Phanerozoic may contain actual residual carbon-14, and that the level of carbon-14 may be estimated by the data given by Beukins (1993). This explanation assumes that the amount of carbon-14 in coal is reliably estimated at 0.014 pmc (95% confidence limits 0.0025 to 0.044 pmc). [10] This fits with the creationist predictions noted above. It still assumes less than careful technique in most of the experiments, but not to quite the same degree as the hypothesis of complete contamination. Differentiating this hypothesis from that of complete contamination would probably require measuring very pure carbon from coal, using perhaps the method of Beukins (1993), and comparing it with carbon-12 and carbon-13 separated from any possible contamination by carbon-14 using a mass spectrometer. One would have to be meticulous in one's technique, and use the largest masses possible.
A third explanation of the above data is that the data given by Beukins (1992) are approximately correct and that higher measured levels of carbon-14 in "old" carbon represent contamination. The 14C/C ratio for carbon in coal is then in the range of 0.052 to 0.12 pmc. The upper value is within the limits of error of several of the lower measurements for coal. It is also within range of the measurements on 200 Ma old graphite by Nakai et al. (1984). It fits the estimate of contamination/residual activity of Kirner et al. (1997). The only measurements lower than this on possibly fossil material are those on coal tar by Farwell et al. (1984), and calcium carbide and methane by Beukins (1993). The result obtained by Farwell et al. does not have a reported standard deviation, and so it may not be in serious conflict with this hypothesis. The data on calcium carbide by Beukins is in conflict, but should not be determinative until it is reproduced, especially considering the wide confidence limits. The major challenge to this hypothesis is the data on methane by Beukins. If this is reproducible and theoretically sound, it would indicate that a substantial proportion of natural gas has no carbon-14. However, until similar results are obtained for coal, fossil shells, or other definitively fossil material, it cannot destroy the hypothesis that fossil material has significant amounts of carbon-14. Of the hypotheses outlined above, I find this third hypothesis to present the case for explaining the data at present.
Hypotheses which propose that there is less than a given level of carbon-14 in a given fossil material should predict that, with the proper care, we can find fossil material that measures less than that level. On the other hand, hypotheses which propose that there is a certain level of carbon-14 in a given fossil material should predict that, with the proper care, we can measure less than that level with other carbon (for example, from a mass spectrometer), and not with the fossil material in question. It will be interesting to follow the results of future experiments.
There is one other possibility that has not been discussed yet. The pre-Flood biosphere may not have been in equilibrium, or even pseudo-equilibrium. It is possible that the atmosphere was not as well mixed as at present, and that various reservoirs of carbon may have had different 14C/C ratios. Thus the data noted by Brown (1988) and even that reported by Snelling (1997, 1998, 1999) may not be in error. Rather, we may be seeing a spectrum of activity. This hypothesis is also a short-age hypothesis. It has not been discussed, not so much because it cannot be correct, but because it makes no specific testable predictions. It does predict that somewhere there should be residual carbon-14 in antediluvian samples, but it does not predict that any given specimen should have a measurable percentage of carbon-14.
RESIDUAL ACTIVITY WOULD ELIMINATE AN AGE GREATER THAN 100,000 YEARS FOR LIFE ON EARTH
The existence of truly residual carbon-14 in material that has been assigned an age greater than 300,000 years would invalidate long-age theories. As noted above, any specimen of greater age than 300,000 years should have less than 1 atom of carbon-14 per gram of carbon. If the entire earth were made of nothing but carbon-14, all but one atom would decay to nitrogen-14 in 1 million years, and that atom would have a greater than 99% chance of also decaying. In 2 million years the weight of the entire known universe in carbon-14 could decay to nitrogen. Thus if there is residual activity in material considered to be 350 million years old, or 2 million years old, or even 300,000 years old, the material in question simply is not that old. In view of our previous discussion, it is probably not even 100,000 years old.
It is interesting to follow the implications of the data further. Since it is believable that most fossil carbon has roughly the same 14C/C ratio, it is reasonable to conclude that all this carbon was in the biosphere at approximately the same time. In that case, since most, if not all, fossil carbon was deposited by water, the data suggest a flood of massive proportions, and that the biblical account has to be taken seriously. If the difference between fossil carbon and Precambrian carbon is approximately 0.05 pmc, and we assume that 0.05 pmc is the true level of residual carbon-14 in pre-Flood fossil carbon, then the first simplistic approximation to the time of burial of fossil carbon is 19,000 years ago. A reasonable upper limit for the time of burial is 25,000 years ago, and with favorable assumptions regarding the pre-burial 14C/C ratio, a time of burial as recent as 4,300 years ago (the traditional Masoretic date for the Flood) is not unreasonable from these considerations alone.
FURTHER STUDY CAN PROVIDE MORE EVIDENCE FOR THE AGE OF LIFE ON EARTH
The data we have at present, although they are most easily interpreted as against a long age for life on Earth, cannot prove a short age. Even more data cannot prove either a short or a long age. First, there are legitimate questions that can be raised about any data, present or future. Proof is elusive in science. It will always remain possible that the available data may be interpreted another way, or is inaccurate. For someone who doubts a short age for life on Earth on other empirical grounds, those doubts may outweigh the positive evidence noted above, or even outweigh further experimental evidences, although at some point the accumulated evidence regarding this phenomenon should outweigh other evidence if it is sufficiently corroborated.
Second (and less legitimately), if a short (or a long) age for life on Earth is philosophically ruled out, no amount of evidence matters. The entire exercise of science then degenerates into an attempt to find evidence to support one's philosophical position, and science ceases to be a search for truth. Then the above data are not allowed to teach anything, and are simply utilized for the sake of argument, or else discounted in an attempt to prevent their use by someone with an opposing view.
For anyone who is seriously considering both a long age and a short age of life on Earth, the above data support the latter and argue against the former. Additional experiments may further support a short age, or change that picture. In either case further experiments can become important, as they help one make an important choice in one's worldview.
ENDNOTES
[1]Readers unfamiliar with carbon-14 dating may find it helpful to consult my previous article on the subject (Giem 1997b), or to consult a general introduction, such as Geyh and Schleicher 1990, p 162-180.
[2]It is theoretically possible to propose that life has been here for 1-4 billion years and that radioactive decay constants have not remained constant during that time. However, this position would require that one accept a timescale based on mechanistic and uniformitarian assumptions while denying those assumptions. I do not know of anyone who seriously proposes such a theory.
[3]The mathematics are as follows: If we call the original 14C/C ratio R0, and use a maximum R0 of 10 (compared to the "modern" [1850] 14C/C ratio), and if we call the half-life of carbon-14 h, and use the most accurate h (5,730 years) for our calculations, and if we express the results R in pmc, then the formula for a given sample is
R = 100% × 2(-t/h) × R0
= 1000% × 2(-t/5730 years) .
For 100,000 years, R = 5.577×10-3 pmc. For 200,000 years, R = 3.111×10 -8 pmc, and for 300,000 years, R = 1.735×10-13 pmc. Since in a gram of ordinary carbon at "present" there are 5×10 12 atoms of carbon-14, in a 300,000-year-old specimen there should be 0.009 atoms of carbon-14 per gram of carbon.
[4]Coal is obviously largely fossil material. Natural gas may or may not be (see Planetary Sciences Unit 1982). Evidence for petroleum being of fossil origin would include optically active compounds and compounds usually derived from organisms.
[5]Equilibrium with the biosphere means that there is enough exchange of carbon between the biosphere and the given material that the 14C/C ratio in the given material is the same as that in the biosphere.
[6]Some predictions of machine background include >100,000 radiocarbon years (<0.0004 pmc) (Muller 1977), less than 1 count per run (>50,000-60,000 years) (Nelson et al. 1977), less than 1 count per day (Doucas et al. 1978), and >70,000 radiocarbon years (<0.016 pmc) (Bennett et al. 1977).
[7]In some experiments, only the total energy of the particles (and their position) is measured. In this case there is some overlap between the tail of the carbon-13 peak and the carbon-14 peak. In these experiments, there is more uncertainty about the background.
[8]The values were published in Kirner et al. (1997). The standard deviation of the smaller value is from Taylor (personal communication 2000). The standard deviation (or more precisely standard error) of the larger value varies considerably depending on whether it is calculated experimentally or from theoretical standard deviations of the measurements. I do not have enough measurements at present to give a precise value, but estimates would range from 0.001 to 0.02 pmc.
[9]The data from before 1979 are summarized in Giem (1997a, p 184). They are not plotted as they are all on graphite or dolomite.
[10]If the distribution is a Poisson distribution, the 95% confidence limits are 0.0025 to 0.044 pmc. However, if the uncertainty is largely due to overlap of carbon-14 counts with the tail of carbon-13 and/or carbon-12 counts, the 95% confidence limits should be roughly 0.0 to 0.034. This is possible. Some laboratories only test one of the properties of carbon-14 to distinguish it from carbon-13, in which case there can be some overlap between the two.
REFERENCES
Aardsma GE. 1991. Radiocarbon and the Genesis flood. El Cajon, CA: Institute for Creation Research.
Aerts-Bijma AT, Meijer HAJ, van der Plicht J. 1997. AMS sample handling in Groningen. Nuclear Instruments and Methods in Physics Research B 123:221-225.
Arnold M, Bard E, Maurice P, Duplessy JC. 1987. 14C dating with the Gif-sur-Yvette tandetron accelerator: status report. Nuclear Instruments and Methods in Physics Research B 29:120-123.
Bennett CL, Beukins RP, Clover MR, Gove HE, Liebert RB, Litherland AE, Purser KH, Sondheim WE. 1977. Radiocarbon dating using electrostatic accelerators: negative ions provide the key. Science 198:508-510.
Beukins RP. 1990. High-precision intercomparison at Isotrace. Radiocarbon 32:335-339.
Beukins RP. 1992. Radiocarbon accelerator mass spectrometry: background, precision, and accuracy. In: Taylor RE, Long A, Kra RS, editors. Radiocarbon After Four Decades: An Interdisciplinary Perspective. NY: Springer-Verlag, p 230-239.
Beukins RP. 1993. Radiocarbon accelerator mass spectrometry: background and contamination. Nuclear Instruments and Methods in Physics Research B 79:620-623.
Beukins RP, Gurfinkel DM, Lee HW. 1992. Progress at the Isotrace Radiocarbon Facility. Radiocarbon 28:229-236.
Bonani G, Hofmann H-J, Morenzoni E, Nessi M, Suter M, Wölffi W. 1986. The ETH/SIN Dating Facility: a status report. Radiocarbon 28:246-255.
Brown RH. 1979. The interpretation of C-14 dates. Origins 6:30-44.
Brown RH. 1988. The upper limit of C-14 age? Origins 15:39-43.
Brown RM, Andrews HR, Ball CC, Burn N, Davies WG, Imahori Y, Milton JCD, Workman W. 1983. Recent 14C measurements with the Chalk River tandem accelerator. Radiocarbon 25:701-710.
Donahue DJ, Beck JW, Biddulph D, Burr GS, Courtney C, Damon PE, Hatheway AL, Hewitt L, Jull AJT, Lange T, Lifton N, Maddock R, McHargue LR, O'Malley JM, Toolin LJ. 1997. Status of the NSF-Arizona AMS laboratory. Nuclear Instruments and Methods in Physics Research B 123:51-56.
Donahue DJ, Jull AJT, Toolin LJ. 1990. Radiocarbon measurements at the University of Arizona AMS facility. Nuclear Instruments and Methods in Physics Research B 52:224-228.
Donahue DJ, Jull AJT, Zabel TH. 1984. Results of radioisotope measurements at the NSF-University of Arizona tandem accelerator mass spectrometer facility. Nuclear Instruments and Methods in Physics Research B 5:162-166.
Doucas G, Garman EF, Hyder HRMcK, Sinclair D, Hedges REM, White NR. 1978. Detection of 14C using a small van de Graaff accelerator. Nature 276:253-255.
Farwell GW, Grootes PM, Leach DD, Schmidt FH. 1984. The accelerator mass spectrometry facility at the University of Washington: current status and an application to the 14C profile of a tree. Nuclear Instruments and Methods in Physics Research B 5:144-149.
Florkowski T, Morawska L, Rozanski K. 1988. Natural production of radionuclides in geological formations. Nuclear Geophysics 2:1-14.
Geyh MA, Schleicher H. 1990. Absolute age determination: physical and chemical dating methods and their application. Newcomb RC, translator. Berlin: Springer-Verlag.
Giem PAL. 1997a. Scientific theology. Riverside, CA: La Sierra University Press.
Giem PAL. 1997b. Carbon-14 dating methods and experimental implications. Origins 24:50-64.
Gillespie R, Hedges REM. 1984. Laboratory contamination in radiocarbon accelerator mass spectrometry. Nuclear Instruments and Methods in Physics Research B 5:294-296.
Grootes PM, Mook WG, Vogel JC, de Vries AE, Haring A, Kistemaker J. 1975. Enrichment of radiocarbon for dating samples up to 75,000 years. Zeitschrift für Naturforschung 30a:1-14.
Grootes PM, Stuiver M, Farwell GW, Leach DD, Schmidt FH. 1986. Radiocarbon dating with the University of Washington accelerator mass spectrometry system. Radiocarbon 28:237-245.
Gulliksen S, Thomsen MS. 1992. Estimation of background contamination levels for gas counting and AMS target preparation in Trondheim. Radiocarbon 34:312-317.
Gurfinkel DM. 1987. An assessment of laboratory contamination at the Isotrace radiocarbon facility. Radiocarbon 29:335-346.
Jull AJT, Donahue DJ, Hatheway AL, Linick TW, Toolin LJ. 1986. Production of graphite targets by deposition from CO/H 2 for precision accelerator 14C measurements. Radiocarbon 28:191-197.
Kirner DL, Burky R, Taylor RE, Southon JR. 1997. Radiocarbon dating organic residues at the microgram level. Nuclear Instruments and Methods in Physics Research B 123:214-217.
Kirner DL, Taylor RE, Southon JR. 1995. Reduction in backgrounds of microsamples for AMS 14C dating. Radiocarbon 37:697-704.
Kitagawa H, Masuzawa T, Makamura T, Matsumoto E. 1993. A batch preparation method for graphite targets with low background for AMS 14C measurements. Radiocarbon 35:295-300.
Kretschmer W, Anton G, Benz M, Blasche S, Erler E, Finckh E, Fischer L, Kerscher H, Kotva A, Klein M, Leigart M, Morgenroth G. 1998. The Erlangen AMS facility and its applications in 14C sediment and bone dating. Radiocarbon 40:231-238.
McNichol AP, Gagnon AR, Osborne EA, Hutton DL, Von Reden KF, Schneider RJ. 1995. Improvements in procedural blanks at NOSAMS: reflections of improvements in sample preparation and accelerator operation. Radiocarbon 37:683-691.
Middleton R, Klein J, Fink D. 1989. A CO2 negative ion source for 14C dating. Nuclear Instruments and Methods in Physics Research B 43:231-239.
Muller RA. 1977. Radioisotope dating with a cyclotron. Science 196:489-494.
Nakai N, Nakamura T, Kimura M, Sakase T, Sato S, Sakai A. 1984. Accelerator mass spectroscopy of 14C at Nagoya University. Nuclear Instruments and Methods in Physics Research B 5:171-174.
Nelson DE, Korteling RG, Stott WR. 1977. Carbon-14: direct detection at natural concentrations. Science 198:507-508.
Nelson DE, Vogel JS, Southon JR, Brown TA. 1986. Accelerator radiocarbon dating at SFU. Radiocarbon 28:215-222.
Pearson A, McNichol AP, Schneider RJ, Von Reden CF. 1998. Microscale AMS 14C measurements at NOSAMS. Radiocarbon 40:61-75.
Planetary Sciences Unit, University of Cambridge. 1982. Mantle methane — fool's gold? Nature 300:312-313.
Scharpenseel HW, Becker-Heidmann P. 1992. Twenty-five years of radiocarbon dating soils: paradigm of erring and learning. Radiocarbon 34:541-549.
Schleicher M, Grootes PM, Nadeau M-J, Schoon A. 1998. The carbonate 14C background and its components at the Leibniz AMS facility. Radiocarbon 40:85-93.
Schmidt FH, Balsley DR, Leach DD. 1987. Early expectations of AMS: greater ages and tiny fractions. One failure? — One success. Nuclear Instruments and Methods in Physics Research B 29:97-99.
Snelling A. 1997. Radioactive 'dating' in conflict! Fossil wood in ancient lava flow yields radiocarbon. Creation Ex Nihilo 20(1):24-27.
Snelling A. 1998. Stumping old-age dogma: radiocarbon in an 'ancient' fossil tree stump casts doubt on traditional rock/fossil dating. Creation Ex Nihilo 20(4):48-51.
Snelling A. 1999. Dating dilemma: fossil wood in ancient sandstone. Creation Ex Nihilo 21(3):39-41.
Terrasi F, Campajola L, Brondi A, Cipriano M, D'Onofrio A, Fioretto E, Romano M, Azzi C, Bella F, Tuniz C. 1990. AMS at the TTT-3 tandem accelerator in Naples. Nuclear Instruments and Methods in Physics Research B 52:259-262.
Van der Borg K, Alderliesten C, de Jong AFM, van den Brink A, de Haas AP, Kersemaekers HJH, Raaymakers JEMJ. 1997. Precision and mass fractionation in 14C analysis with AMS. Nuclear Instruments and Methods in Physics Research B 123:97-101.
Van der Plicht J, Aaerts A, Wijma S, Zondervan A. 1995. First results from the Groningen AMS Facility. Radiocarbon 39:657-661.
Vogel JS, Nelson DE, Southon JR. 1987. 14C background levels in an accelerator mass spectrometry system. Radiocarbon 29:323-333.
Wild E, Golser R, Hille P, Kutschera W, Priller A, Puchegger S, Rom W, Steier P. 1998. First 14C results for archaeological and forensic studies at the Vienna environmental research accelerator. Radiocarbon 40:273-281.
Zito R, Donahue DJ, Davis SN, Bentley HW, Fritz P. 1980. Possible subsurface production of carbon-14. Geophysical Research Letters 7(4):235-238.
COVER PICTURE
 Coal seams in the Cretaceous Star Point Sandstone north of Price, Utah. The article by Paul Giem discusses the possible implications of radiocarbon dates of less than 100,000 years that have been found in some coals. Photographs courtesy of Clyde L. Webster — higher resolution version (242K)
Coal seams in the Cretaceous Star Point Sandstone north of Price, Utah. The article by Paul Giem discusses the possible implications of radiocarbon dates of less than 100,000 years that have been found in some coals. Photographs courtesy of Clyde L. Webster — higher resolution version (242K)