©Copyright 2018 GEOSCIENCE RESEARCH INSTITUTE
11060 Campus Street • Loma Linda, California 92350 • 909-558-4548

Editorial Note: This blog was first written as an article for Geoscience Reports (n.14) in Spring 1992.
More details about chaos theory can be found at:
- Oxford English Dictionary --- Chaos Theory: The branch of mathematics that deals with complex systems whose behavior is highly sensitive to slight changes in conditions, so that small alterations can give rise to strikingly great consequences.
- Wikipedia
- Britannica
- Fractal Foundation
Recent references on this topic can be found at:
- Deep Simplicity: Bringing Order to Chaos and Complexity / April 2005 / John Gribbin / Random House
- Chaos: A Very Short Introduction / April 2007 / Leonard Smith / Oxford University Press
- Chaos and Dynamical Systems / August 2019 / David Feldman / Princeton University Press
More details about the new physics can be found at:
- Wikipedia --- quantum mechanics
- Can Science Explain It All? / 1991 / Benjamin L. Clausen / College and University Dialogue 3(2):8-10. --- strengths and weaknesses of science, wave/particle nature of light, relativity, quantum mechanics, limitations of science, scientific revolutions
- Quantum Mechanics: the Strange World at Small Dimensions / 1991 / B. L. Clausen / Origins 18(1): 39-47. --- models, quantum mechanics, wave/particle duality, radioactive decay, Einstein philosophy
- How Final Is Final? / 1995 / Benjamin L. Clausen / Origins 22(1): 43-46. --- naturalism, reductionism, complexity, god-of-the-gaps, quantum mechanics, Newtonian mechanics, mechanistic clockwork universe, theodicy, beauty
- Teaching Physical Science from a Christian Perspective / 2002 / Ben Clausen / Journal of Adventist Education, 64(5): 22-28. --- Christian founding fathers of science, trend toward naturalism, objectivity, determinism, reductionism, design, time, origins, the end
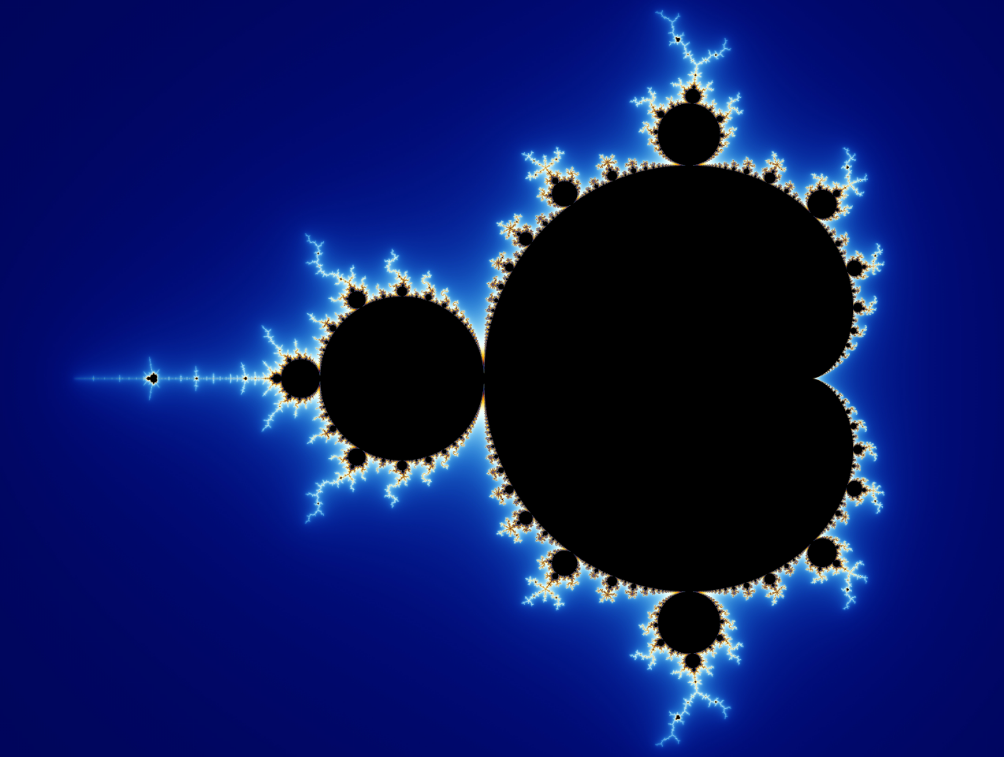
The Mandelbrot set illustrates a complex and beautiful system resulting from a very simple rule. More details can be found here and here.
Image created by Wolfgang Beyer with the program Ultra Fractal 3, available at https://commons.wikimedia.org, licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license.New developments in science that come under the general heading of "chaos" have been described as revolutionary and the basis for a genuinely new paradigm. It has brought about the realization that instead of understanding most of nature in principle, science has really addressed only the very restricted subset of phenomena that can be analyzed by simple methods. Chaos has had a broad impact, influencing mechanics, astronomy, solid-state physics, ecology, meteorology, biology, and many other areas. For those interested in a popular book on the subject, Chaos: Making a New Science (Gleick, 1987) is probably the best, with Fractals in Your Future (Lewis, 1991) being particularly appropriate for teaching high school level students. At a slightly more advanced level is Fractals, Chaos, Power Laws: Minutes from an Infinite Paradise (Schroeder, 1991).
To understand the revolutionary nature of chaos requires some history. In the 1600s Newton developed classical mechanics to describe the motions of bodies in our solar system. In the 1800s Laplace extended the ideas of classical mechanics to suggest determinism---the motion of every body in the universe is completely determined. As a result, "[u]niversal, immutable laws, absolute precision, and strict predictability were ideas that were habitually believed to characterize science." Newtonian physics assumed "that the initial data determine the future, unambiguously, uniquely, and forever ... [and] that the qualitative aspects of the motion are not too sensitive to the precise initial data." Newton's laws could be solved exactly for 2 bodies, such as the sun and the earth. For a system containing more than 2 bodies, such as the earth-moon-sun system, accurate approximations were used. "More involved problems were---so was the common belief---only technically different from the special examples." Newtonian physics was a dramatic success in predicting the return of Halley's comet in 1757 after its observation in 1682, and in predicting a new planet (Neptune) based on the observed irregularities in the orbit of Uranus. "These ideas were so successful and suggestive that they stimulated the search for similar laws in all of physics (and most of science)." (Dresden, 1992).
Unfortunately "the general attitude toward classical physics was based on an uncritical, unanalyzed acceptance of the ideology of Newtonian physics." "It is not clear whether physicists and astronomers were aware of the tenuous mathematical basis of their (often unspoken) beliefs, but it is pretty clear that they didn't worry too much about it" (Dresden, 1992). At the turn of the last century Poincaré in his The New Methods of Celestial Mechanics wondered about the stability of the solar system. He "discovered that with even the very smallest perturbation, some orbits behaved in an erratic, even chaotic way." This was true even for a closed system, that should be particularly amenable to analysis by classical mechanics. Quantum mechanics and the Heisenberg uncertainty principle yielded indeterminacy in another area a few years later, and Poincaré's ideas were forgotten for a time. "Small wonder, since even Poincaré had abandoned the ideas, saying, 'These things are so bizarre that I cannot bear to contemplate them.'" Poincaré's ideas have only come to the forefront again in the last 15 years or so. (Briggs and Peat, 1989).
Now classical mechanics is being re-evaluated. It has been found that "properties were inferred from the detailed examinations of very few examples---maybe five or six altogether." "[A]ll the general features attributed to classical mechanics are in general wrong. The exactly soluble examples are not generic; they are in fact quite atypical." "[C]haotic behavior, contrary to earlier beliefs, was a rather general property and not a pathological feature of some contrived system," and even relatively simple systems can exhibit frightfully complex behavior. (Dresden, 1992)
For chaotic systems, a slight imprecision results in indeterminism. This slight imprecision may result from uncertainty in the initial data, or from approximate calculations based on perturbations of an exact solution. For such systems, predictive errors develop exponentially with time, and an initial small imprecision eventually results in total loss of predictability. (Davies, 1990)
Examples of chaotic behavior are numerous. The famous Butterfly Effect suggests "that a butterfly stirring the air today in Peking can transform storm systems next month in New York" (Gleick, 1987). Population dynamics of rabbits can be affected in unpredictable ways by small changes in food supply. Earthquakes, snow avalanches, and dinosaur extinctions have been studied using chaos methods. One simple experiment involved adding sand to a sand pile on a four-centimeter plate, one grain at a time. Sand avalanches would occur after as few as one or as many as several thousand grains were added to the pile. The addition of a sand grain would cause an avalanche in unpredictable ways. (Bak and Chen, 1991)
Chaos theory brings attention to the fact that errors in describing the future based on present approximate data in classical mechanics develop exponentially with time.
Ben Clausen, PhD
Geoscience Research Institute
References
Bak, Per and Kan Chen. 1991. Self-Organized Criticality. Scientific American 264(1):46-53.
Briggs, John and F. David Peat. 1989. Turbulent Mirror. Harper & Row, NY, pp.26-29.
Davies, Paul. 1990. Chaos frees the Universe. New Scientist 128(1737):48-51.
Dresden, Max. 1992. Chaos: A New Scientific Paradigm---or Science by Public Relations? The Physics Teacher 30(1):10-14.
Gleick, James. 1987. Chaos: Making a New Science. Viking Penguin, NY.
Lewis, Ron S. 1991. Fractals in Your Future. Media Magic, Nicasio, CA.
Schroeder, Manfred. 1991. Fractals, Chaos, Power Laws: Minutes from an Infinite Paradise. Freeman, NY.
Questions for reflection:
- Does chaos theory disprove determinism?
- Are complex systems deterministic? Are chaotic systems deterministic?
- Can order come out of chaos?
- Could it be that errors in backwards extrapolation from present earth history data also develop exponentially with time and less is known about the past than previously realized?